The Black-Scholes Model, pillar of the modern financial system
The Black-Scholes Model stands as one of the cornerstones of modern finance, revolutionizing the way financial derivatives are priced and traded. Developed by Fischer Black, Myron Scholes, and Robert Merton in the early 1970s, this mathematical framework provided a breakthrough in understanding options pricing and paved the way for advancements in financial engineering and risk management. This essay explores the evolution, applications, and potential future improvements of the Black-Scholes Model.
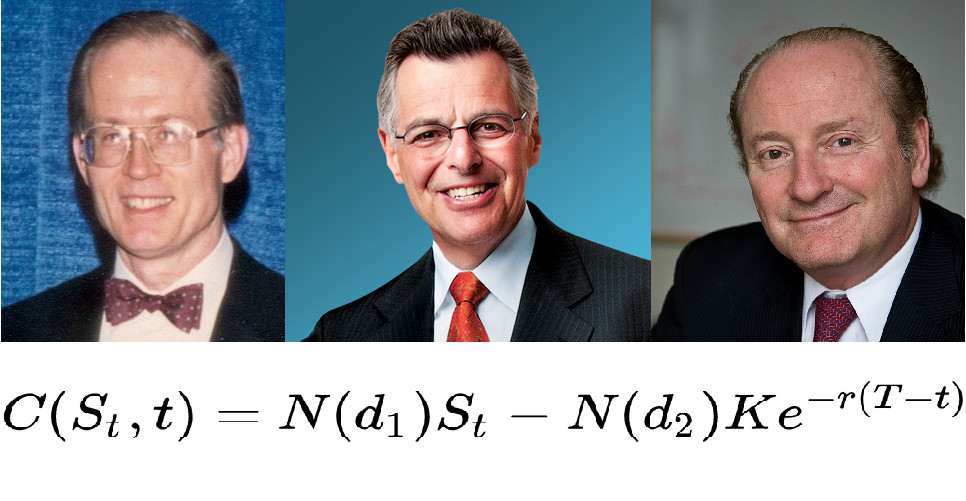
Evolution of the Black-Scholes Model:
The Black-Scholes Model emerged at a crucial juncture in financial history when the options market was burgeoning, but lacked a comprehensive theoretical framework for pricing options. By combining insights from stochastic calculus, probability theory, and finance, Black and Scholes introduced a groundbreaking equation that could accurately determine the fair value of European-style options.
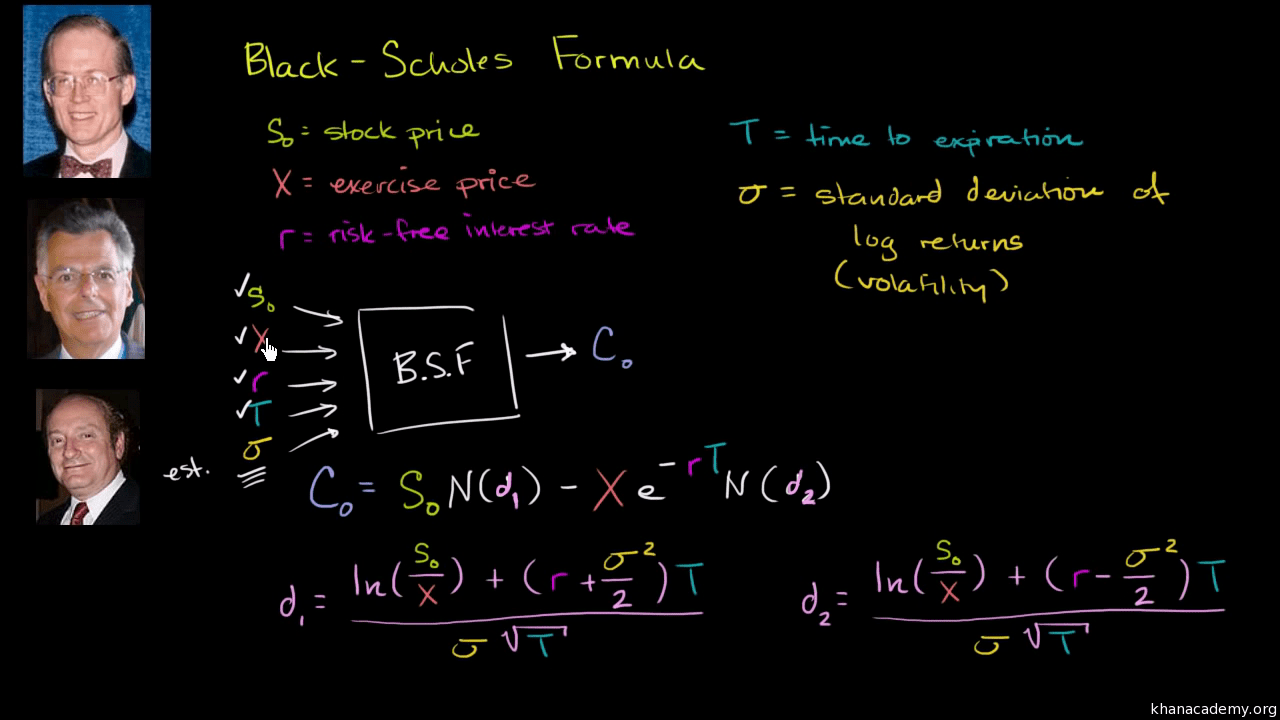
The model's key assumptions include the efficient market hypothesis, continuous trading, constant risk-free rate, and log-normal distribution of stock prices. Despite its simplifying assumptions, the Black-Scholes Model provided a practical tool for traders and investors to quantify the risk and return associated with options trading.
Applications of the Black-Scholes Model:
The Black-Scholes Model has found widespread applications across various domains within finance:
Options Pricing: The model's primary application lies in pricing European call and put options, providing traders and investors with insights into the fair value of these derivative securities.
Risk Management: Financial institutions and portfolio managers utilize the Black-Scholes Model to hedge their options exposure, thereby mitigating risk and optimizing portfolio performance.
Arbitrage Strategies: Traders leverage the model to identify mispriced options and execute arbitrage strategies, thereby capitalizing on market inefficiencies.
Corporate Finance: The Black-Scholes Model is instrumental in valuing employee stock options (ESOs) and other equity-based compensation plans, facilitating effective decision-making by corporate executives and shareholders.
Academic Research: The model has spurred extensive academic research in the fields of mathematical finance, economics, and quantitative analysis, contributing to the development of advanced derivative pricing models and risk management techniques.
Future Improvements of the Black-Scholes Model:
While the Black-Scholes Model has revolutionized the options market, it is not without limitations. Some of the key areas for potential future improvements include:
Incorporating Market Frictions: The model assumes frictionless markets, which may not reflect the complexities of real-world trading environments. Future enhancements could integrate transaction costs, liquidity constraints, and market impact into the pricing framework.
Accounting for Jump Diffusions: The Black-Scholes Model assumes continuous price movements, overlooking the possibility of abrupt price jumps. Enhancements could incorporate jump diffusion processes to better capture market dynamics, especially during periods of heightened volatility.
Volatility Smile/Skew: The model assumes constant volatility across all strike prices and maturities, disregarding the empirically observed volatility smile/skew phenomenon. Future iterations could incorporate more sophisticated volatility surfaces to better reflect market realities.
Extensions to Exotic Options: While the model is well-suited for pricing standard European options, it may not adequately capture the complexities of exotic options with non-standard features. Future improvements could focus on developing models tailored to exotic derivatives, such as barrier options, Asian options, and lookback options.
Behavioral Finance Considerations: The model assumes rational behavior and risk neutrality among market participants, which may not align with the principles of behavioral finance. Future enhancements could integrate behavioral biases and investor sentiment into the pricing framework to improve predictive accuracy.
Incorporating AI calculations: AI can enhance the Black-Scholes model in various ways. It aids in data analysis, predicting market trends, and managing risks associated with options trading. Through refining option pricing, facilitating high-frequency trading, and optimizing portfolio management, AI augments decision-making processes in financial markets, ultimately improving accuracy and efficiency.
In conclusion, the Black-Scholes Model represents a landmark achievement in financial theory, providing a powerful framework for options pricing and risk management. While the model has proven instrumental in shaping modern finance, ongoing research efforts aim to address its limitations and develop more robust pricing models that better reflect real-world market dynamics. As financial markets continue to evolve, the quest for improved models and methodologies remains essential in facilitating informed decision-making and fostering innovation in the field of quantitative finance.
